[Edit 2013-10: The existence of this post has bothered me since I first published it three years ago. Basically, this was intended as the first installment of what was to have been a sprawling series of posts attempting to reconcile what I will loosely term "Eastern monism" with the semantically precise, mathematical ontology I have been trying to develop in light of hexagonal principles—and in a real, meaningful way, not just in some fake-ass pop quantum mysticism sense. I won't recapitulate my whole line of thought here, but suffice to say I had and continue to have more substantive and I think relevant ideas on these matters than those expounded in this limited post, which by itself I think ends up conveying either that I misunderstand or misinterpret people like Watts, or that I am not particularly interested in the finer implications of what they tried to communicate. And I would like to think neither is true. Watts was a huge intellectual influence on me in my youth, and it was writings such as his that actually turned me from my earlier phase of hexagonal thinking as a teenager—only to return to it with more clarity of thought over a decade later. And having later become of aware of his passing references to hexagons, I was very eager to draw out these ideas and sort of riff on them with the greater perspective gained from the ensuing years. But I left the task unfinished, and, again, I think this post, by itself, is sort of weird. I gave serious thought to deleting it or leaving it unpublished in this latest iteration of the site, but ultimately decided to leave it here, with this disclaimer. I intend to revisit these issues at some point, hopefully in greater detail, at which point I will perhaps modify or edit this post further.]
"Where is it writ large that talking monkeys should be able to model the cosmos?" – Terence McKenna
 I remember reading a short but eloquent endorsement of hexagons by Alan Watts once, probably seven or eight years ago. Since I wasn't particularly interested in hexagons at the time, I quickly forgot about it, and where exactly I had read it. However, times have changed and my hexagonal thinking has evolved, and with the advent of Google Books and all, I recently decided to look into the matter further. After an exhaustive search, I found two mentions of hexagons in Watts' books, which I shall now share with you, and duly expound upon.
I remember reading a short but eloquent endorsement of hexagons by Alan Watts once, probably seven or eight years ago. Since I wasn't particularly interested in hexagons at the time, I quickly forgot about it, and where exactly I had read it. However, times have changed and my hexagonal thinking has evolved, and with the advent of Google Books and all, I recently decided to look into the matter further. After an exhaustive search, I found two mentions of hexagons in Watts' books, which I shall now share with you, and duly expound upon.
I am not entirely sure either of these is in fact the passage I remember reading, but if it were either of them it would be this first one, which can be found in the essay What on Earth Are We Doing?, from the collection Cloud-Hidden, Whereabouts Unknown:

 I remember reading a short but eloquent endorsement of hexagons by
I remember reading a short but eloquent endorsement of hexagons by  The last components necessary to make this site passably acceptable to me were completed last evening. Thus, as promised, on time and under budget, I hereby declare this site to exist. Several important facts to keep in mind:
The last components necessary to make this site passably acceptable to me were completed last evening. Thus, as promised, on time and under budget, I hereby declare this site to exist. Several important facts to keep in mind:

 I seem to have taken an unintentional hiatus from putting my ass into this project, due largely to the fact that it has of late been too hot to move, think, or do anything at all really. Come hell or high water though, I will "launch" this site by the first of Sextilis, being actually the fifteenth (13th;) anniversary of the launch of my first Hexnet site, back in 1995. That is probably an unnecessarily dramatic theme to tie in with this whole procedure, but it seems silly to allow such an auspicious date to pass unobserved. Also, I really need to get on with this. I mean, I have numerous medium-term goals to tweak the layout—I want to clean up and expand the links section, among other things—but most of that can wait. I am really just trying to wrap up a bit of library content, actually. Which at this point seems fairly silly, since I long ago gave up on my original idea of having some sort of centralized "Hexagonal Manifesto" or what have you, in favor of a more modular if somewhat disjointed collection of one-off content items and such. Anyway, since 1 August falls lamentably on a Sunday this year, which is a terrible day to do anything PR-wise (as is the entire month of August, really), I expect to do some sort of formal what have you by next week some time. Or you know whenever.
I seem to have taken an unintentional hiatus from putting my ass into this project, due largely to the fact that it has of late been too hot to move, think, or do anything at all really. Come hell or high water though, I will "launch" this site by the first of Sextilis, being actually the fifteenth (13th;) anniversary of the launch of my first Hexnet site, back in 1995. That is probably an unnecessarily dramatic theme to tie in with this whole procedure, but it seems silly to allow such an auspicious date to pass unobserved. Also, I really need to get on with this. I mean, I have numerous medium-term goals to tweak the layout—I want to clean up and expand the links section, among other things—but most of that can wait. I am really just trying to wrap up a bit of library content, actually. Which at this point seems fairly silly, since I long ago gave up on my original idea of having some sort of centralized "Hexagonal Manifesto" or what have you, in favor of a more modular if somewhat disjointed collection of one-off content items and such. Anyway, since 1 August falls lamentably on a Sunday this year, which is a terrible day to do anything PR-wise (as is the entire month of August, really), I expect to do some sort of formal what have you by next week some time. Or you know whenever.

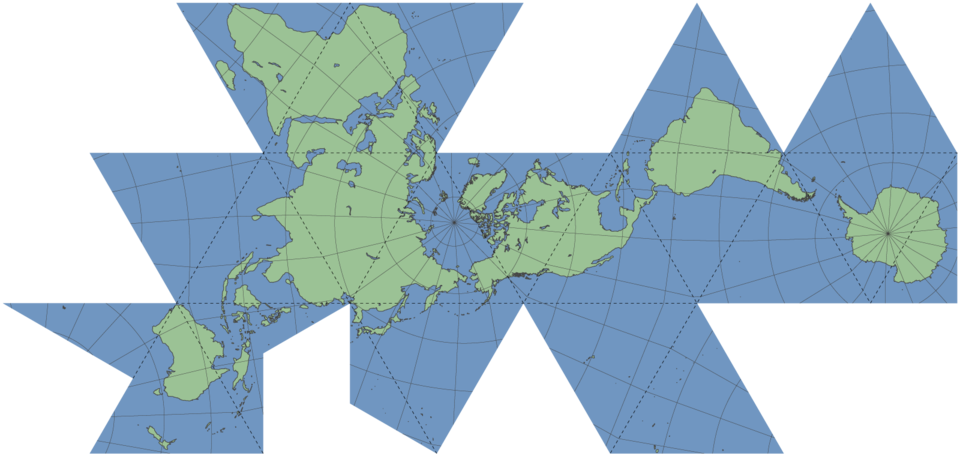
 Anyone following the hexagonal news of late has no doubt noticed a flurry of stories related to the upcoming release of
Anyone following the hexagonal news of late has no doubt noticed a flurry of stories related to the upcoming release of 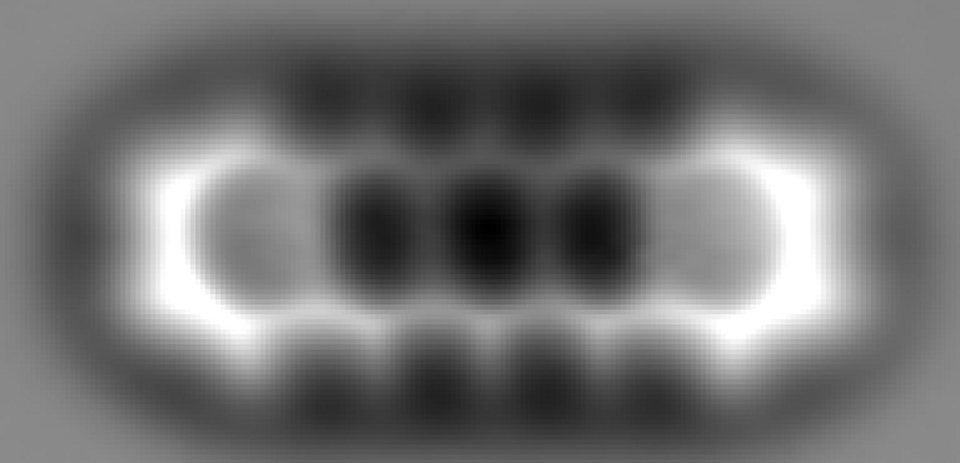
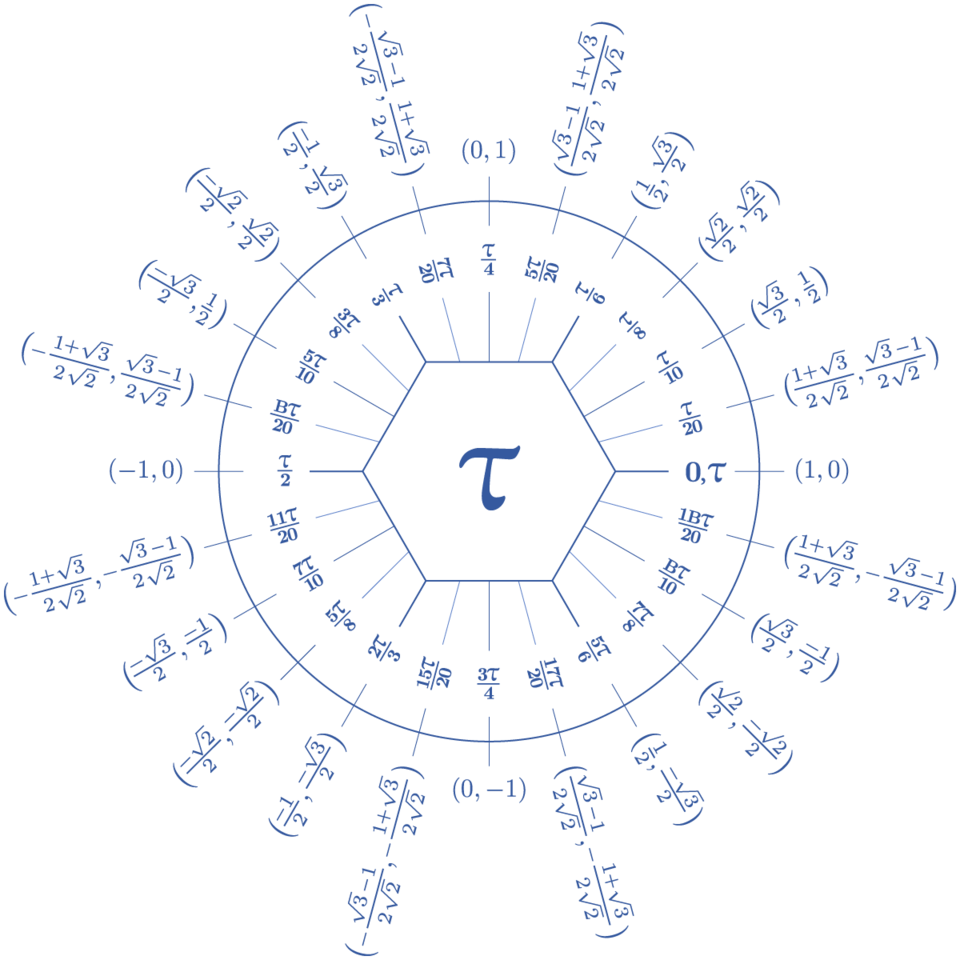
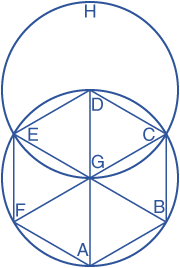 Let ABCDEF be the given circle. It is required to inscribe an equilateral and equiangular hexagon in the circle ABCDEF.
Let ABCDEF be the given circle. It is required to inscribe an equilateral and equiangular hexagon in the circle ABCDEF.
 I have been reading about the
I have been reading about the 
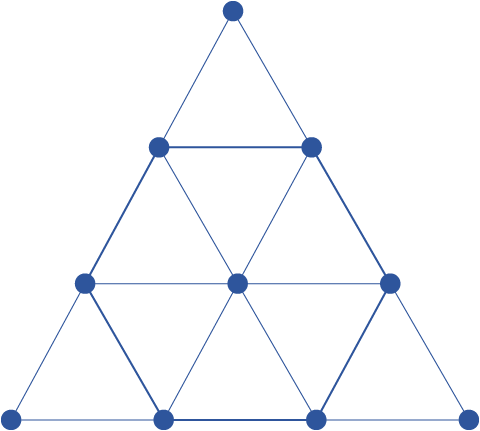
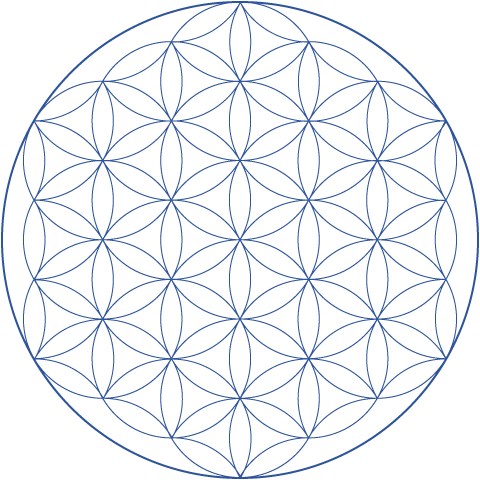
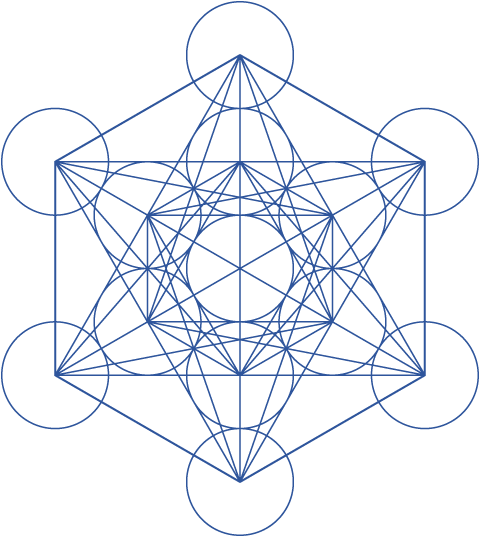


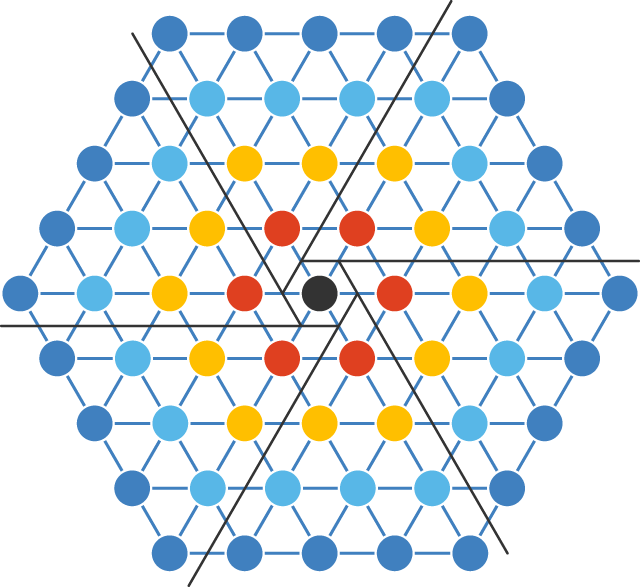
 It looks like the hexagonal zeitgeist is finally floating to the surface of our collective media environment. Two stories in particular off of our
It looks like the hexagonal zeitgeist is finally floating to the surface of our collective media environment. Two stories in particular off of our 


 This site is not yet completed, and at least one core content piece is still in progress, but after some months of halfassery, I am prepared at this time to move into Phase I of my three-phase site launch program. This site is live as of May 30, 2010 (May 26, 11B6), and hopefully shall remain so. FTVW.
This site is not yet completed, and at least one core content piece is still in progress, but after some months of halfassery, I am prepared at this time to move into Phase I of my three-phase site launch program. This site is live as of May 30, 2010 (May 26, 11B6), and hopefully shall remain so. FTVW.



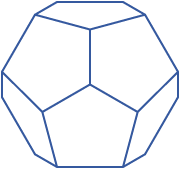 I have been completely unable to find any sort of followup to this since 2003. The whole idea may have been discarded by now, I'm not sure, but considering that Plato speculated—for no particular reason modern science is aware of—that the universe was shaped like a dodecahedron nigh doz. 1,500 years ago, this is obviously a significant conjecture to put forward.
I have been completely unable to find any sort of followup to this since 2003. The whole idea may have been discarded by now, I'm not sure, but considering that Plato speculated—for no particular reason modern science is aware of—that the universe was shaped like a dodecahedron nigh doz. 1,500 years ago, this is obviously a significant conjecture to put forward.