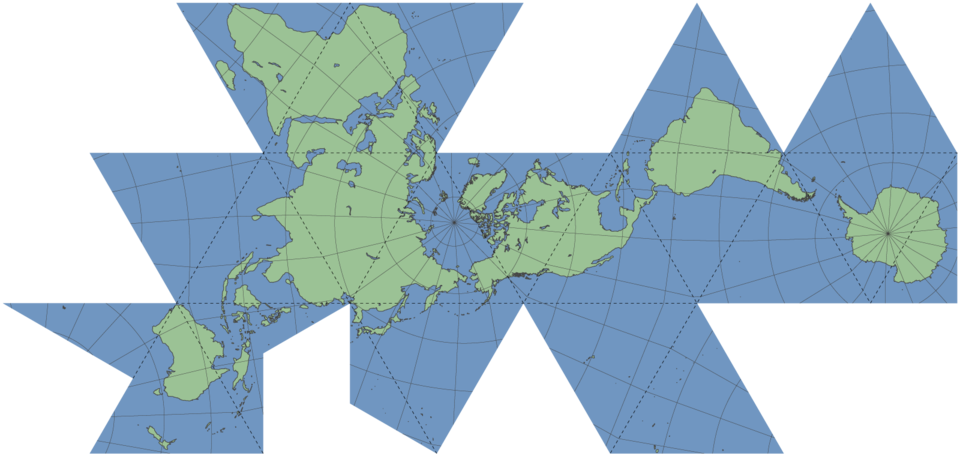
Here we see the well-known icosahedral version of R. Buckminster Fuller's dymaxion map projection of the earth's surface. A similar projection can be created by mapping the globe onto any other convex polyhedron, and unfolding it into a flat net of polygons. This particular icosahedral net has become widely used due to its relatively contiguous and undivided treatment of the earth's land mass, which for general cartographic purposes is often seen as useful. However, other nets—icosahedral and otherwise—exist that accentuate and prioritize other aspects of the earth's surface, such as the oceans.
The icosahedron provides the most accurate approximation of the surface of a sphere among the five regular polyhedra. Higher-sided convex polyhedra such as the truncated icosahedron, &c., can provide a more accurate projection of the globe (and may indeed be useful in other applications), but in practice it is difficult to conceptualize a two-dimensional map consisting of dozens of polygons dangling off in all directions, particularly when land masses and other points of interest are divided up among various non-contiguous faces. As five triangles come together at each vertex of a {3,5} regular icosahedron, and six triangles come together (in a hexagonal manner!) at each vertex of a Euclidean tessellation of triangles, up to five triangles (but as few as one) can and do share common vertices when the net of this particular map is flattened out. This, although it no doubt looks a bit disjointed compared to conventional oval or rectangular projections, produces a much more "solid" map than many other polyhedral nets would be capable of. Of course, one could also project the map onto nets of cubes or tetrahedra, which could be even more contiguous, but this would be far less accurate a map. The icosahedral net provides an adequate and appropriate balance between viewing convenience and projective accuracy.
The map has a number of noteworthy advantages over other map projections. It has less shape distortion than, for instance, the Mollweide projection, and less size distortion than of course the Mercator projection. All map projections, and particularly whole globe projections, represent compromises between the various distortions introduced by projecting any spheroid surface onto a Euclidean plane. Different projections are useful for different purposes, but in general the dymaxion map produces one of the more accurate and balanced projections of the globe, featuring both relatively accurate shapes and angles of surface features as well as fairly consistent size relations—the only major drawback of course being its somewhat awkward shape. The scale of the dymaxion map is most accurate along the edges of the triangular panels, which correspond to geodesic line segments over the surface of the globe. Features towards the interior of the triangular panels tend to shrink in relation to the edges, but not by a significant amount.
It is worth noting, of course, that the general principles of the icosahedral map projection can be applied to local maps as well—each triangular panel can be subdivided into smaller triangular tessellations, in the manner of a large geodesic dome, which may be locally useful for, say, navigational purposes, which the whole-globe icosahedral net map may not be particularly helpful for. I am neither a cartographer nor a navigator though, so I don't really know.
More information on the dymaxion map, including several other nets and projections, can be found at: Dymaxion map on Wikipedia. The original SVG image on which it is based can be found here on Wikimedia Commons.
This image is based on the above-linked original, created by Eric Gaba, Wikimedia Commons user: Sting, and published under the Creative Commons Attribution-ShareAlike 2.5 license. (The colors have been changed to match this site's color scheme, and I flipped it around 180 degrees, so it looks less obviously copied—I'm crafty like that.) Anyway: you are free to copy, distribute, and transmit the work, and to adapt the work, under the following conditions: You must attribute the work in the manner specified by the author or licensor (but not in any way that suggests that they endorse you or your use of the work). If you alter, transform, or build upon this work, you may distribute the resulting work only under the same or similar license to this one. Et cetera.
 Dymaxion map
Dymaxion map