This is a unit circle diagram using both dozenal notation (as with elsewhere on this site, using "A" and "B" for ten and eleven) and the newly proposed circle constant τ (tau), which is equal to 2π. The advantages of τ over π are numerous and obvious—instead of a full circle of arc being two of anything, it is just one τ. Put another way, τ is simply the number of radians in a circle.
By combining τ with dozenal notation, and dividing the circle into 20; (dec. 24.) equal angles (being the lowest common denominator of 8 and 10; (12.), covering all 45. and 30. degree angles), we see the clear advantages of both systems. Compare to a conventional trigonometric unit circle using decimal notation and π, which—though it may be apprehended with the comfort of established convention—is not really a particularly meaningful way of describing a circle.
For more information on tau as a circle constant, and compelling arguments for its adoption, please consult the following fascinating articles:
- Pi Is Wrong! by Bob Palais
- The Tau Manifesto by Michael Hartl
I spent a disturbing amount of time making this image, and it represents the last time I will attempt to lay out mathematical expressions in Illustrator. However, in all probability this is the first unit circle diagram ever created in the history of mankind using both the tau circle constant (by any name) and dozenal notation. It is my contribution to the future of trigonometry. As with all mathematical diagrams on this site, please feel free to disseminate widely, but of course some credit and a link back to this site would be greatly appreciated—though not required.
[EDIT 2012-09-30: This diagram has now been made into a WATCH by a MAJOR WATCH COMPANY: http://cadencewatch.com/tau-watch. I am in possession of one of these watches. It seems fairly sturdy. Though I'm not really a watch person.]
 Dozenal tau unit circle
Dozenal tau unit circle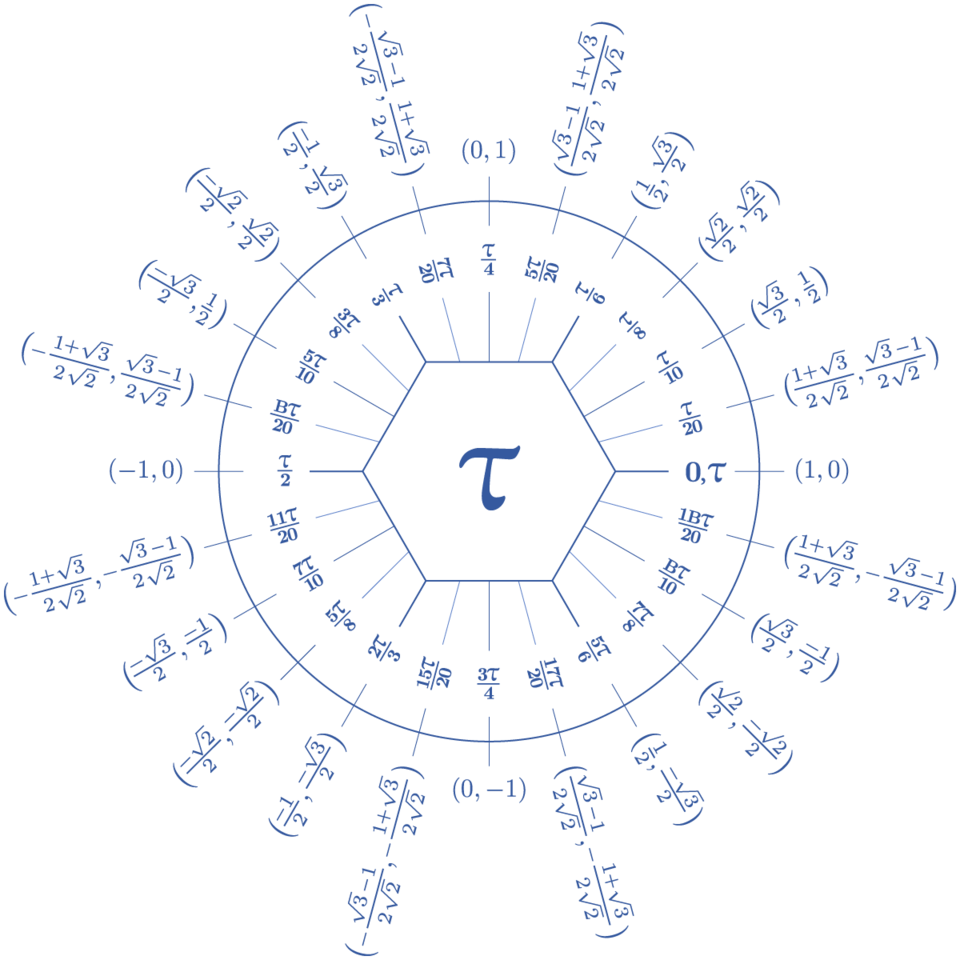
Errors in this Tau unit circle
Hello all, I am new to this concept of using tau over pi and I've been looking into eta as well. The eta unit circle doesn't exist yet, so I decided to make one myself, however, as I was doing some calculation and comparisons with the pi unit circle and the tau unit circle, I noticed that there are some serious errors in the tau unit circle.
Take note on how pi/4 equals tau/8 (which would equal eta/2). When you take a look at the 5tau/20 almost on top of the circle, you can see that 5tau/20 equals 5/20 tau. this equals tau/4. The circle claims to say that 5tau/20 does not equal tau/4.
The same goes for tau/10, which according to the pi unit circle should equal pi/6. To correct that mistake, it should have been tau/12.
pi/6 = 0.523.. tau/10 = 0.628.. tau/10 = 18 degrees This unit circle claims that tau/10 equals 15 degrees.
This tau unit circle is riddled with these mistakes and anybody using this table should mind the errors it contains.
Please correct this unit circle, because I am willing to look into using tau and making some equations next to pi and eta.
Kind regards and greetings from the Netherlands
~Michiel
Re: Errors in this Tau unit circle
I have to assume you just came across this on an image search or something and didn't actually read the title or body or the post. The operative word here—besides "tau"—is of course "dozenal." All numbers are in base twelve (12). It is late, and I am tired, and I don't really have the mental strength for trigonometry at this hour, but I believe this misunderstanding is at the root of your concerns. 10 is "twelve." The numeral glyphs are 0, 1, 2, 3, 4, 5, 6, 7, 8, 9, A, and B in my convention. 10 is twelve, B is eleven, A is ten, and so on.
This is all explained elsewhere but looking back on it now I do see that the post just sort of assumes you know what "dozenal" means. Perhaps I will modify the image to include some sort of in-image advertisement of this fact.
That being said, I've never really had this image fully vetted by any sort of real math person. I showed it to several math types early on (including Mr. Hartl himself), and they all seemed duly impressed with it and said it was beautiful and so forth (which I greatly appreciated), but I do get the feeling that none of them ever looked at it too closely. So there could yet be mistakes in there I don't know. I am reasonably confident of its accuracy at this point though.
I would urge you to take this opportunity to consider the advantages of the dozenal system over decimal. There is some enjoyable literature in our Hexagonal Library on the subject. Thanks for the comment though. I spent a good deal of time making this (as noted in the post), it's good to see it's gotten out there. Even if people are grossly misinterpreting it.
This is great work, consider it swiped! Your math all looks right on to me. Although technically, leaving a radical in the denominator is frowned on, I see how it makes the patterns easier to see, and subsequently memorize if desired.
Inspired by your example, I have drawn (on paper) a similar graph of my own. Mine differs in that it is divided only into twelfths with solid lines and eighths in dotted lines, leaving out some of the lesser-used 2-dozenths.
I also labeled each "spoke" with its dozenal point value of tau. In other words, 30 degrees on standard decimalized chart = Tau/12 on yours = .1Tau on mine. 45 degrees = Tau/8 = .16Tau, 90 degrees = Tau/4 = .3Tau, 300 degrees = 5Tau/6 = .A Tau, 330 degrees = 11/12 = .B Tau, etc. This notation really brings home to me the advantages of both tau and dozenal in the unit circle. As a first attempt, my chart looks like a combination of a clock and a pizza, which doesn't sound appetizing at all, but tastes like dessert to the mind. Looking at it makes everything just about the unit circle "click" for me.
So hey... thanks.
Re: Errors in this Tau unit circle
I'm afraid that you are the one in error. The title states that the circle is the DOZENAL tau unit circle. In accordance with this, 20 does not say two tens, but two dozens, or, in decimal, 24.
5/24, as you can hopefully see, is a twenty-fourth short of 6/24, which would be 1/4.
wrong values
I think I understand the dozenal notation, but the coordinates still seem wrong. For example tau/20 in dozenal = pi/12 in base 10, and neither sin(pi/12) nor cos(pi/12) equal the (x, y) values shown. Instead sin(pi/12) = (sqrt(6) - sqrt(2)) / 4.
Re: wrong values
Thank you for your interest. I am reasonably confident however that the numbers are correct. The expression you provided seems to be equivalent to the one in the diagram, so I'm not sure what the point of disagreement is. What would you contend the numeric value of sin(π/12.) should be?
Re: wrong values
Sorry it's taken me a while to reply. I forgot I left the above comment and just chanced on this page again. I have no idea what I was thinking before, our expressions for sin(pi/12) are indeed equivalent and all the values seem correct to me now.
apologies, a humble student of elementary trig
Re: Errors in this Tau unit circle
I think this is incorrect, but only because you call it dozenal but rather use the duodecimal standard symbols. Of course as a mathematical base the two systems are identical, but the dozenal standard would be to use either ᘔ or X for ten (there's some disagreement I understand, but I reckon the X is more dyslexic-friendly) and Ɛ for eleven, while duodecimal is the decimal-centric convention and doesn't bother adopt unique symbols for its additional digits.
Re: Errors in this Tau unit circle
I continue using "a" and "b" because this is the standard convention used by most programming languages when converting integers to superdecimal strings, and when parsing strings to integers. Given that there is no universally agreed upon glyph set for dozenal notation, I see no reason to complicate my code and my writing with an arbitrarily-chosen numeral set when the alphanumeric system is pretty universally understood already.
Ultimately all dozenal numeral characters should be unique and distinct from the decimal characters, that numbers may be easily differentiated at first glance rather than requiring a semicolon, or something like the 0 or 0x prefixes for octal or hexadecimal, etc. Unil that happens, simply swapping out the two non-decimal numerals doesn't really advance the ball much as far as I'm concerned. I'm aware that this is a minority view among dozenalists, but, again, I see no compelling reason to change, and many reasons not to.
As for your contention vis-a-vis terminology, I have to say I'm not actually aware of this distinction between dozenal and duodecimal. I, like most dozenalists, avoid the term "duodecimal" largely because of its unhelpfully obvious etymology.
Re: wrong values
what is all this nonsense
Re: wrong values
Well, this is proposing two changes to mathematical thinking: using tau instead of pi (tau = 2*pi), and using base 12 instead of base 10. There are convincing arguments for both, and I'm convinced myself on tau's benefits: however, I believe that base 12 counting would cause more confusion than it's worth, what with our current number system based on 10.
Rectified Unit Circle
For all who see this;
I edited it to be more friendly to those just starting trig, it's now in base 10 notation and features only 12 angles (Every angle divisible by 30, so 30, 60, 90, 120, 150 etc).
Find it here:
imgur: http://imgur.com/a/jRAAM slimg: http://sli.mg/8UWXtZ